Three Forms Of Quadratic Equations

Examples of Quadratic Equation

What is a quadratic equation? A quadratic equation is an equation of the second caste, pregnant information technology contains at least i term that is squared. The standard form is ax² + bx + c = 0 with a, b and c being constants, or numerical coefficients, and x being an unknown variable. Continue reading for examples of quadratic equations in standard and non-standard forms, as well as a list of quadratic equation terms.

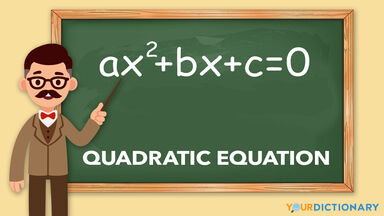quadratic equation example

Standard Grade Equation Examples

The easiest way to larn quadratic equations is to get-go in standard form. While non every quadratic equation you lot run into volition exist in this form, it'due south still helpful to come across examples. Keep in mind that the get-go abiding a cannot be a nothing.

Examples of the standard grade of a quadratic equation (ax² + bx + c = 0) include:

6x² + 11x - 35 = 0
2x² - 4x - two = 0
-4x² - 7x +12 = 0
20x² -15x - x = 0
x² -x - 3 = 0
5x² - 2x - 9 = 0
3x² + 4x + ii = 0
-10² +6x + 18 = 0

Incomplete Quadratic Equation Examples

As yous develop your algebra skills, you'll discover that not every quadratic equation is in the standard form. Bank check out examples of several dissimilar instances of non-standard quadratic equations.

Missing the Linear Coefficient

Sometimes a quadratic equation doesn't have the linear coefficient or the bx function of the equation. Examples include:

2x² - 64 = 0
x² - 16 = 0
9x² + 49 = 0
-2x² - 4 = 0
4x² + 81 = 0
-ten² - 9 = 0
3x² - 36 = 0
6x² + 144 = 0

Missing the Constant Term

Quadratic equations can also lack the constant term, or c. For example:

x² - 7x = 0
2x² + 8x = 0
-ten² - 9x = 0
x² + 2x = 0
-6x² - 3x = 0
-5x² + x = 0
-12x² + 13x = 0
11x² - 27x = 0

Quadratic Equation Examples in Factored Form

Factoring is one style to solve a quadratic equation. Here are examples of quadratic equations in factored form:

(x + 2)(x - 3) = 0 [standard form: ten² - 1x - 6 = 0]
(x + ane)(x + 6) = 0 [standard form: ten² + 7x + 6 = 0]
(x - 6)(x + 1) = 0 [standard grade: 10² - 5x - half-dozen = 0]
-three(10 - 4)(2x + 3) = 0 [standard grade: -6x² + 15x + 36 = 0]
(x − five)(x + 3) = 0 [standard form: x² − 2x − 15 = 0]
(x - 5)(10 + 2) = 0 [standard course: x² - 3x - 10 = 0]
(x - 4)(x + 2) = 0 [standard class: 10² - 2x - eight = 0]
(2x+iii)(3x - 2) = 0 [standard class: 6x² + 5x - 6]

Examples of Quadratic Equations in Other Forms

Examples of quadratic equations in other forms include:

x(x - two) = 4 [upon multiplying and moving the four, becomes 10² - 2x - 4 = 0]
x(2x + 3) = 12 [upon multiplying and moving the 12, becomes 2x² - 3x - 12 = 0]
3x(x + 8) = -2 [upon multiplying and moving the -2, becomes 3x² + 24x + 2 = 0]
5x² = nine - ten [moving the 9 and -10 to the other side, becomes 5x² + x - 9]
-6x² = -2 + 10 [moving the -2 and x to the other side, becomes -6x² - ten + 2]
ten² = 27x -14 [moving the -fourteen and 27x to the other side, becomes x² - 27x + 14]
x² + 2x = 1 [moving "1" to the other side, becomes x² + 2x - 1 = 0]
4x² - 7x = 15 [moving 15 to the other side, becomes 4x² + 7x - 15 = 0]
-8x² + 3x = -100 [moving -100 to the other side, becomes -8x² + 3x + 100 = 0]
25x + vi = 99 ten² [moving 99 xⁱⁱ to the other side, becomes -99 x² + 25x + 6 = 0]

Quadratic Equation Terms

If you'd similar a lilliputian more than caption on quadratic equations, cheque out a list of essential math vocabulary terms. They can assist you lot understand more almost quadratic equations, what they're for and how to solve them.

coefficient - the number that multiplies the variable past a specific amount (the 4 in 4x)
completing the square - method to solve a quadratic equation in which you divide all terms by the coefficient and add or decrease the constant terms
constant - a stock-still value in an equation
caste - the largest exponent in an equation; the ten² makes an equation a quadratic equation (or "2d degree"), while x ³would make an equation a cubic equation (or "third degree")
discriminant - the gene in a quadratic equation (b² - 4ac in the quadratic formula) that indicates if the equation has a real number solution
factoring - method of solving a quadratic equation by looking for the multiplication factors of the equation
parabola - a plane curve which is a graphed representation of a quadratic function
quadratic equation - an equation where the highest exponent of a variable is a square (ten² )
quadratic function - equation expressed as f(10) = a(10 - h)two which is used to graph a parabola
real number - numbers that have a positive result when multiplied by itself (as opposed to an imaginary number, which has a negative result [√(−1)]
variable - the letter in a mathematical expression that represents an unknown value (usually what you solve for)
vertex - the minimum or maximum value of a graphed quadratic office equally represented past the point where a parabola changes direction
quadratic formula - formula used to solve quadratic equations:

The Logical World of Math

Understanding quadratic equations is a foundational skill for both algebra and geometry. Now that you lot've seen several examples of quadratic equations, yous're well on your mode to solving them! Learn more than about important math skills with these examples of standard deviation and how it's used in statistics.

center schoolhouse
high school
college